previous <<==>> next
PICKETT & ECKEL N4-ES ( 10" / 25 cm Scales )
Enjoy the nice N4-SIMULATION found in the Web ...
http://antiquark.com/sliderule/sim/n4es/virtual-n4es.html
PICKETT & ECKEL INC., Chicago, ILL. / USA (1959)
*******************************************************************
VERSION (B)
S C A L E S of the » ALL METAL VECTOR LOG LOG « Model N4-ES
===================================================================
Front Side [ inverse / RED ] Back Side
===================================================================
3rdRoot(X< 1;4;7 Digits,.. >) LL1 10^0.001X;
3rdRoot(X< 2;5;8 Digits,.. >) [LL-1] [ 1/10^0.001X ]
3rdRoot(X< 3;6;9 Digits,.. >) LL2 10^0.01X;
[LL-2] [ 1/10^0.01X ]
DF πX DF/M X/M
-------------------------------------------------------------------
CF πX CF/M X/M
[CIF] [1/πX] TH <0.1 .. 3> TanH(X)
T1 tan(0.1X) SH1 <0.1 .. 0.88> SinH(0.1X)
T2 tan(X) SH2 <0.88 .. 3> SinH(X)
ST arc(0.01X) LN <0 .. 2.3> lnX
S sin(0.1X); [cos(0.1X)] L <0 .. 1.0> lgX
[CI] [1/X] [CI] [1/X]
C X C X
-------------------------------------------------------------------
D X D X
[DI] [1/X] LL3 10^0.1X;
[LL-3] [ 1/10^0.1X ]
sqrt(X < ODD-No-Digits > ) LL4 10^X;
sqrt(X < EVEN-No-Digits > ) [LL-4] [ 1/10^X ]
There are 2 V E R S I O N S of the » N4-ES « in the collection:
===================================================================
Both have the print "COPYRIGHT 1959" on the sliders,
but d i f f e r e n t LOGOS, END-PIECES & CURSORS ...
Version (A)
|
Version (B)
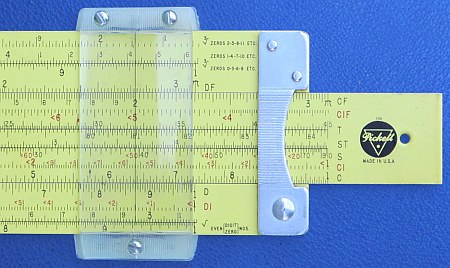R E M A R K S :
===================================================================
This model is made of aluminum. It came with a leather case, which
can be clipped on the user's belt - typical for the USA: As seen in
the "Western Movies" ready for a "quick draw".
This slide rule has the typical "Pickett-Yellow" body: The "-ES" in
the model number means "Eye-Saver"; Models with the "-T"-Extension
(for "Traditional") have a white body.
With 34 scales it was one of the most sophisticated slide rules!
Using the "syncro" LOG-LOG-Scales:
The LogLog scales are designed as a uniquely, split long scale.
Because LL+/- are reciprocal, 2. & .5 (eg.) share the same tick.
The DF/M-Scale is folded at 1/M where e = 2.71828 = EulerConst.
M = lg(e) = 0.43429 and 1/M = ln(10) = 2.30259 IT FOLLOWS:
Using the D-Scale X, the LL-Scales result in Power-of-10( X )
Using the DF/M-Scale X, the LL-Scales result in Power-of-e( X )
Using the LL-Scales X, the D-Scale result in lgX
Using the LL-Scales X, the DF/M-Scale result in lnX
Using the Hyperbolic Functions:
Start with value on the SH scales ==>> reading SinH on C scale,
start with value on the C scale ==>> reading ArcSinH on SH.
Start with value on the TH scale ==>> reading TanH on C scale,
start with value on the C scale ==>> reading ArcTanH on TH.
There is no CosH(X) scale; make use of the definition:
TanH(X) = SinH(X)/CosH(X) ==>> CosH(X) = SinH(X)/TanH(X)
Example: CosH(0.345) = 1.060
---------------------------------
With C and D indices coinciding, set the indicator over X on the SH
scale. Move slide until X on the TH scale is under the hairline.
Read CosH(X) on the D scale under the C index.